Plasticity
Plasticity Theory
The constitutive theory is about relating stress $\sigma$ and strain $\varepsilon$.
The total strain $\varepsilon$ can be decomposed into
\[\varepsilon = \varepsilon^p + \varepsilon^e\]
The constitutive relation must characterize the relation between the stress $\varepsilon$ for both $\varepsilon^p$ and $\varepsilon^e$ . The constitutive law for $\varepsilon^e$ is linear, i.e.,
\[\sigma = C\varepsilon^e\Leftrightarrow\varepsilon^e = C^{-1}\sigma\]
The constitutive law for $\varepsilon^p$ is described by an ordinary differential equation
\[\boxed{\dot\varepsilon^p_{ij} = \phi h_{ij}}\tag{1}\]
Here $h_{ij}$ may arise from a potential function $g(\sigma, \xi)$, where $\xi$ is called internal variables
\[h_{ij} = \frac{\partial g}{\partial \sigma_{ij}}\]
and $\phi$ is a scalar function of the form
\[\phi = \eta(\sigma,\xi) f(\sigma, \xi)_+ := \eta(\sigma,\xi)\max(0, f(\sigma, \xi))\]
where $f$ is the yield surface.
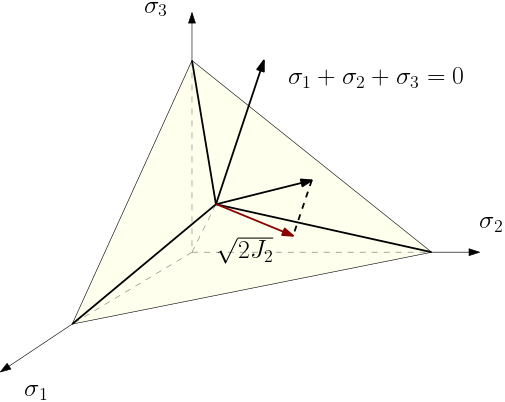
The Tresca yield surface is given by
\[f(\sigma, \xi) = \frac{1}{4}(|\sigma_1-\sigma_2|+|\sigma_2-\sigma_3|+|\sigma_3-\sigma_1|)-k(\xi)\]
In $J_2$ plasticity, we have
\[g(\sigma, \xi) = J_2(\sigma) - k(\xi)^2\]
Thus we have
\[\dot\varepsilon^p_{ij} = \mathrm{const}\times\left(1-\frac{k(\xi)}{J_2(\sigma)}\right)_+ s_{ij}\]
In the rate-indpendent plasticity, there exists $\lambda$ such that
\[\phi = \dot\lambda\]
Since both sides of (1) has derivatives with respect to time, it is time scale independent. That's why we call it rate-independent. Then the flow rule requires that
\[\boxed{\dot \lambda f = 0\quad \lambda\geq 0\quad f\leq 0}\tag{2}\]
If $f<0$ (the yield surface is not hit), the inelasticity strain is not "active"; once $f=0$ is hit, the material reacts by increasing plasticity strain (since $\dot \lambda$ can be nonzero).
The deformation theory studies how $g$ is related to $\sigma$ and $\xi$. If $h$ directs along the outward normal of the yield surface, we can the consequent $g$ the normality rule. For example, $J_2$ plasticity can be formulated is subjected to normality rule. A particular case where normality rule holds is $g=f$, in which case we call (1) the associated flow rule with the yield surface.
Finally, the dynamics of internal variable is given by
\[\boxed{\dot\xi_\alpha = \tilde h_\alpha(\sigma, \xi)}\tag{3}\]
The three equations (1), (2) and (3) fully characterizes the constitutive relation of $\varepsilon^p$.
Numerical Example
| Description | Displacement Field | Vertical Displacement |
|---|---|---|
| Plasticity | 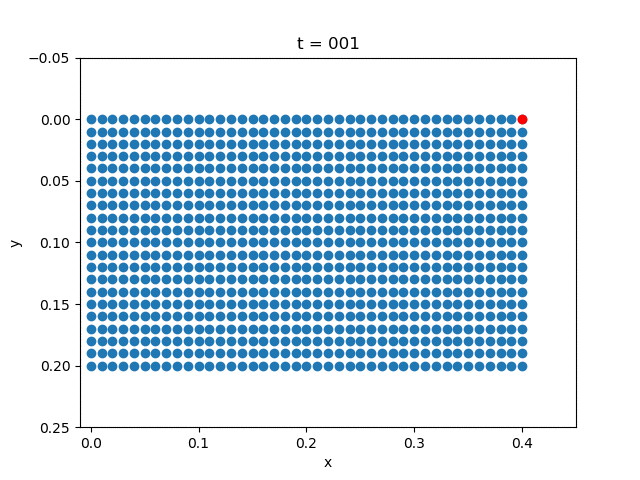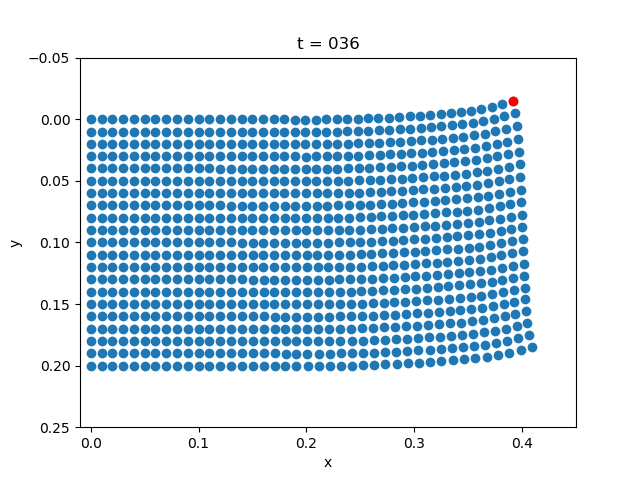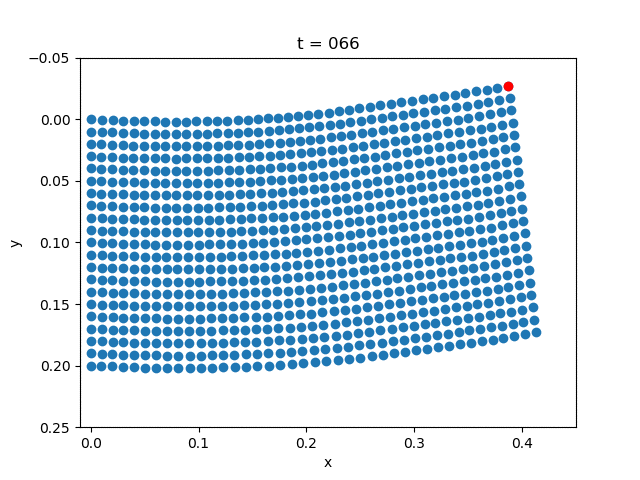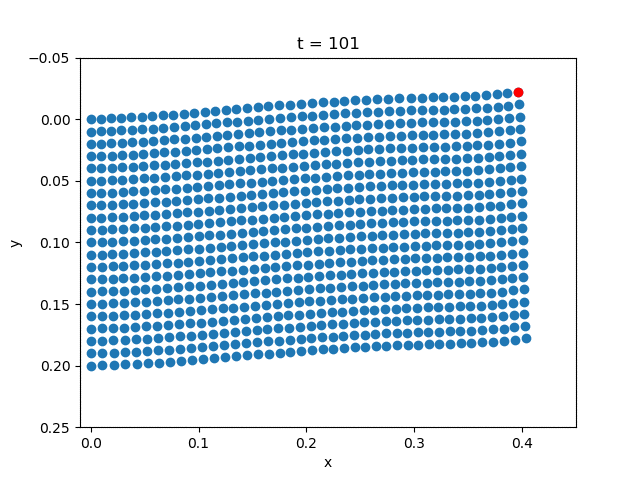 | 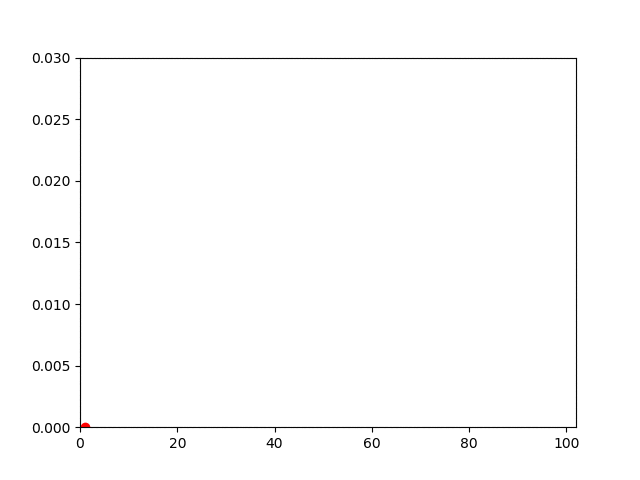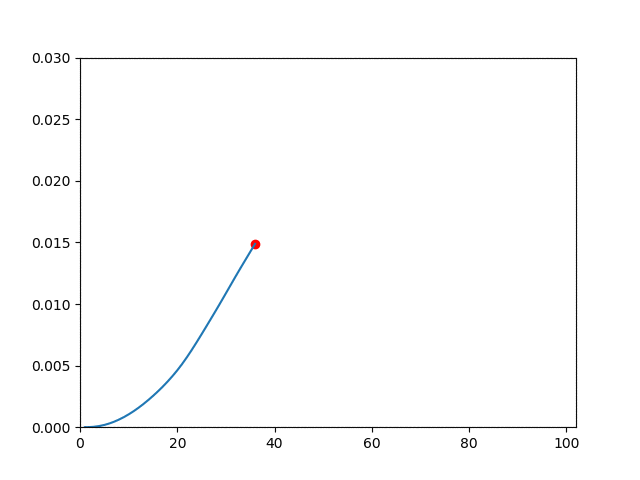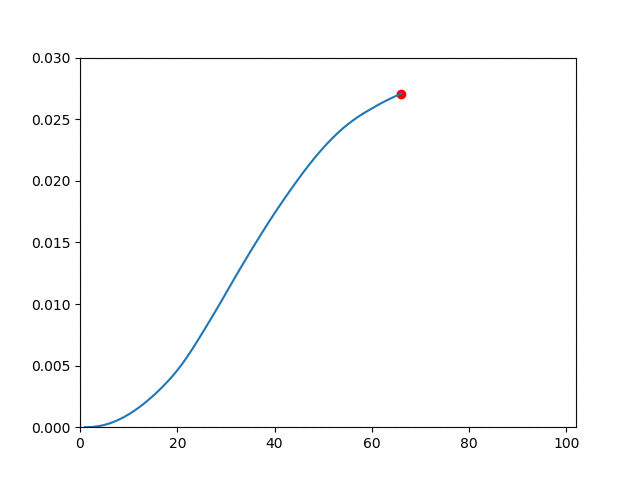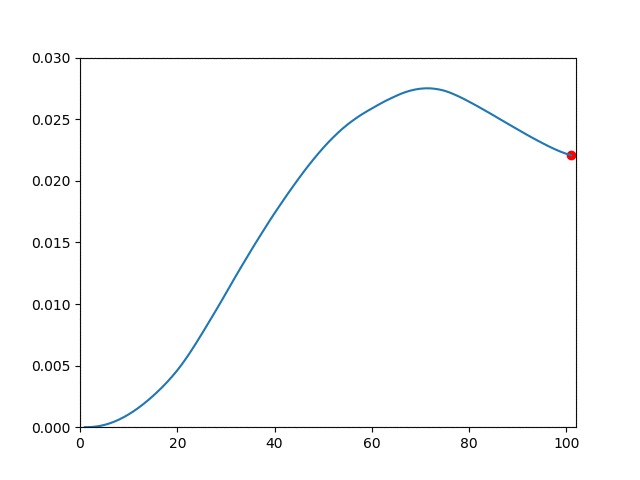 |
| Elasticity |  |  |
using Revise
using AdFem
using SparseArrays
using LinearAlgebra
using PyPlot
αm = 0.0
αf = 0.0
β2 = 0.5*(1 - αm + αf)^2
γ = 0.5 - αm + αf
m = 40
n = 20
h = 0.01
NT = 100
Δt = 1/NT
bdedge = []
for j = 1:n
push!(bdedge, [(j-1)*(m+1)+m+1 j*(m+1)+m+1])
end
bdedge = vcat(bdedge...)
bdnode = Int64[]
for j = 1:n+1
push!(bdnode, (j-1)*(m+1)+1)
end
M = compute_fem_mass_matrix1(m, n, h)
S = spzeros((m+1)*(n+1), (m+1)*(n+1))
M = [M S;S M]
H = diagm(0=>[1,1,0.5])
K = 0.1
σY = 0.03
# σY = 1000.
state = zeros(2(m+1)*(n+1),NT+1)
velo = zeros(2(m+1)*(n+1),NT+1)
acce = zeros(2(m+1)*(n+1),NT+1)
stress = zeros(NT+1, 4*m*n, 3)
internal_variable = zeros(NT+1, 4*m*n)
t = 0.0
for i = 1:NT
@info i
##### STEP 1: Computes the external force ####
T = eval_f_on_boundary_edge((x,y)->0.02*sin(2π*i*Δt), bdedge, m, n, h)
# T = eval_f_on_boundary_edge((x,y)->0.0, bdedge, m, n, h)
T = [zeros(length(T)) -T]
T = compute_fem_traction_term(T, bdedge, m, n, h)
f1 = eval_f_on_gauss_pts((x,y)->0., m, n, h)
f2 = eval_f_on_gauss_pts((x,y)->0., m, n, h)
# f2 = eval_f_on_gauss_pts((x,y)->0.1, m, n, h)
F = compute_fem_source_term(f1, f2, m, n, h)
fext = F+T
##### STEP 2: Extract Variables ####
u = state[:,i]
∂∂u = acce[:,i]
∂u = velo[:,i]
ε0 = eval_strain_on_gauss_pts(u, m, n, h)
σ0 = stress[i,:,:]
α0 = internal_variable[i,:]
##### STEP 3: Newton Iteration ####
global t += (1 - αf)*Δt
∂∂up = ∂∂u[:]
iter = 0
while true
iter += 1
# @info iter
up = (1 - αf)*(u + Δt*∂u + 0.5 * Δt^2 * ((1 - β2)*∂∂u + β2*∂∂up)) + αf*u
global fint, stiff, α, σ = compute_planestressplasticity_stress_and_stiffness_matrix(
up, ε0, σ0, α0, K, σY, H, m, n, h
)
res = M * (∂∂up *(1 - αm) + αm*∂∂u) + fint - fext
A = M*(1 - αm) + (1 - αf) * 0.5 * β2 * Δt^2 * stiff
A, _ = fem_impose_Dirichlet_boundary_condition(A, bdnode, m, n, h)
res[[bdnode; bdnode .+ (m+1)*(n+1)]] .= 0.0
Δ∂∂u = A\res
∂∂up -= Δ∂∂u
err = norm(res)
# @info err
if err<1e-8
break
end
end
global t += αf*Δt
##### STEP 3: Update State Variables ####
u += Δt * ∂u + Δt^2/2 * ((1 - β2) * ∂∂u + β2 * ∂∂up)
∂u += Δt * ((1 - γ) * ∂∂u + γ * ∂∂up)
stress[i+1,:,:] = σ
internal_variable[i+1,:] = α
state[:,i+1] = u
acce[:,i+1] = ∂∂up
velo[:,i+1] = ∂u
end
x = []
y = []
for j= 1:n+1
for i = 1:m+1
push!(x, (i-1)*h)
push!(y, (j-1)*h)
end
end
for i = 1:5:NT+1
close("all")
scatter(x+state[1:(m+1)*(n+1), i], y+state[(m+1)*(n+1)+1:end, i])
scatter(x[m+1]+state[m+1, i],
y[m+1]+state[(m+1)*(n+1)+m+1, i], color="red")
xlabel("x")
ylabel("y")
k = string(i)
k = repeat("0", 3-length(k))*k
title("t = $k")
ylim(-0.05,0.25)
xlim(-0.01, 0.45)
gca().invert_yaxis()
savefig("u$k.png")
close("all");
plot(1:i, -state[(m+1)*(n+1)+m+1, 1:i])
xlim(0, NT+2)
ylim(0, 0.03)
scatter(i, -state[(m+1)*(n+1)+m+1, i], color="red")
savefig("du$k.png")
end
run(`convert -delay 10 -loop 0 u*.png plasticity_u.gif`)
run(`convert -delay 10 -loop 0 du*.png plasticity_du.gif`)